Armstrong numbers, also called narcissistic numbers, are special numbers in math that have a unique property making them fun to explore. They belong to number theory, a branch of mathematics that studies numbers and their relationships. Named after Michael F. Armstrong, these numbers are interesting because of their rare and special characteristic. Understanding Armstrong numbers can be a great way for beginners and students to get a deeper appreciation of mathematics and the amazing patterns that can be found within it. This exploration can spark curiosity and interest in more complex mathematical concepts.
Definition
An Armstrong number is a number that is equal to the sum of its digits, each raised to the power of the number of digits. This means if you take each digit in the number, raise it to the power of the total number of digits, and add them up, you get the original number.
Mathematical Expression
To explain it simply, let’s use a number with ‘n’ digits. This number can be written as:
ABC…N = An + Bn + Cn + … + Nn
Here, A, B, C, …, N are the digits of the number and ‘n’ is the number of digits.
Example of Armstrong Numbers
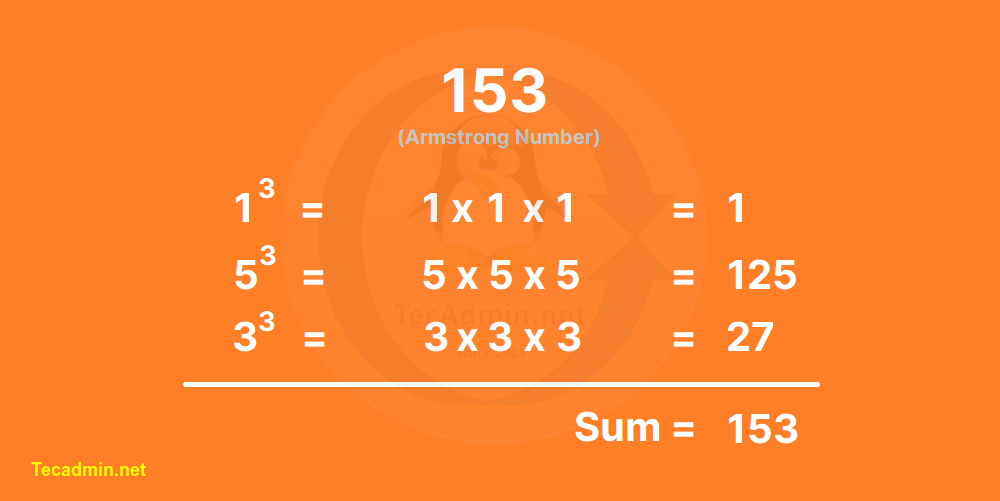
153 is an Armstrong number, also known as a narcissistic number. To determine this, we follow these steps:
- Count the digits:
- 153 has 3 digits.
- Raise each digit to the power of 3 and sum them:
- 13 = 1
- 53 = 125
- 33 = 27
- Sum these values:
- 1 + 125 + 27 = 153
Since the sum of these values equals the original number (153), it confirms that 153 is an Armstrong number.
Other examples of Armstrong numbers are 370, 371, 407, 1634, 8208, and 9474. For example, for the number 371:
33 + 73 + 13 = 27 + 343 + 1 = 371
The sum of each digit to the power of 3 (since there are three digits) equals the original number, so 371 is an Armstrong number.
Properties of Armstrong Numbers
All Armstrong numbers share the same property, but they do not follow a specific pattern, making them hard to list. Some interesting facts about Armstrong numbers are:
- All single-digit numbers are Armstrong numbers: Since there’s only one digit, raising it to the power of 1 gives the original number. For example, the number 5 raised to the power of 1 is 5.
- There are no 2-digit Armstrong numbers: No two-digit number can be written as the sum of the squares of its digits.
- There are four 3-digit Armstrong numbers: These are 153, 370, 371, and 407.
- There are three 4-digit Armstrong numbers: These are 1634, 8208, and 9474.
- Number of digits: The number of n-digit Armstrong numbers decreases as n increases. The largest known Armstrong number has 39 digits.
Conclusion
Armstrong numbers are a fun part of number theory, showing how numbers can have hidden patterns. They captivate the interest of mathematicians, puzzlers, and math enthusiasts. While Armstrong numbers may not have practical applications in daily life, their unique properties remind us of the many fascinating aspects of mathematics. Exploring these numbers can inspire a deeper interest in math and reveal the beauty of numerical patterns. They serve as a gateway to the wider and more complex world of number theory, encouraging learners to dive deeper into mathematical studies.

